What is the largest natural number possible? By using the word natural, I have ruled out the possibility that you simply answer infinity (∞) to torpedo the guessing game. But even if we allowed infinitely large values, this response would cause problems. What about ∞ + 1, ∞2 or ∞∞? If people put forward these replies to the largest natural number question, who would be right?
The answer is no one because infinity is not an ordinary number that follows the usual rules of calculation. For example, the number line is infinite, regardless of whether you start it at –∞, 0 or 1. Therefore, a statement such as ∞ + 1 makes no sense. Furthermore, there are differences even with infinite values: infinity does not always equal infinity. Thus, infinity would not be a guaranteed winner in a largest number contest.
It took humankind several millennia to realize this idea and cast it into a neat theory. At the end of the 19th century, mathematician Georg Cantor laid a foundation for the mathematical concept of infinity by thinking about quantities and their size. For example, {1, 2, 3, 4} and {x, y, z, q} both consist of four elements each and therefore have a size of 4, which experts call a “cardinality of 4.”
On supporting science journalism
If you're enjoying this article, consider supporting our award-winning journalism by subscribing. By purchasing a subscription you are helping to ensure the future of impactful stories about the discoveries and ideas shaping our world today.
The natural numbers {0, 1, 2, 3,...}, on the other hand, contain infinitely many elements. We can add 1 to any natural number; the result is also a natural number. If we now look at the set of all even numbers {0, 2, 4,...}, we might assume that it is just half as large—after all, only every second natural number is contained in it. But Cantor recognized that both sets (the natural and the even numbers) have the same cardinality.
Matching Sets and Countable Infinities
As discussed in another column of mine, Cantor came to this surprising result when he compared the elements of two sets. If you want to find out whether a set A (for example, people at a bus stop) is exactly as large as another set B (free seats on a bus), you can assign an element from B to each element from A. If there are still people left standing at the end, then A is larger than B. If, on the other hand, there are still free seats, then B must be larger than A.
But if you can assign exactly one seat to each person, then both sets are exactly the same size and thus have the same cardinality. In this way, Cantor also studied the cardinality of infinite sets. For example, you can map any natural number to exactly one even number, say, by forming the pairs (0,0), (1,2), (2,4), (3,6),..., (n, 2n). The mapping works out exactly. Neither natural nor even numbers remain at the end. Therefore, both sets contain the same number of elements.
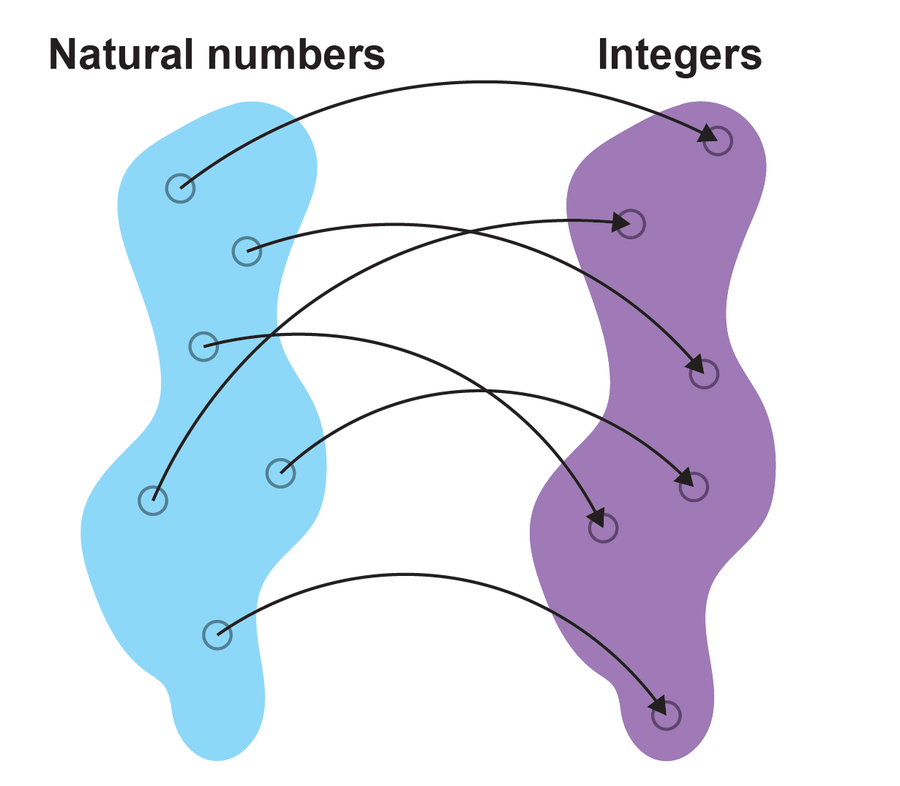
Two sets are equal if there is a one-to-one mapping between the elements of the respective sets. Credit: Spektrum der Wissenschaft/Manon Bischoff, styled by Scientific American
Here an important lesson emerges. When it comes to infinities, do not go with your gut. These ideas are rarely intuitive.
Not only is the cardinality of natural and even numbers the same, the trick with mapping two sets can be applied to other examples as well. For example, the set of all odd numbers is the same size as the set of all natural ones—as is the set of all integers (which include negative values), prime numbers and even the rational numbers (which include fractions). For each of these sets, there is a mapping that uniquely assigns a natural number {1, 2, 3,...} to each element. That means you could—at least theoretically—number the elements of these sets (if you had infinite time and leisure).
The smallest infinity owes its name to this fact. The cardinality of the natural numbers is called “countably infinite” and is represented by ℵ0 (spoken as “aleph zero”). So instead of submitting the answer “infinity” to the largest numbers competition, you could offer ℵ0.
The sets presented so far all have the same cardinality. But real numbers break the pattern. If, in addition to the rational numbers, you also allow irrational values, such as the square root of 2, pi or Chaitin’s constant, then the set suddenly becomes so large that you can no longer enumerate its elements—even if the list is infinitely long.
The Infinity of Real Numbers Exceeds Natural Numbers
Cantor proved this fact with his second “diagonal argument.” This is a proof by contradiction: you start with the assumption that there are countably infinite real numbers and derive a contradictory statement from this idea (that is, “There cannot be countably infinite real numbers”).
To proceed, you do not have to consider all real numbers. It is sufficient to assume that all real values between 0 and 1 are countable (which, we will soon see, is wrong).
Accordingly, you could write all these values in an infinitely long list, with one below the other. For example:
0.32476834567854765 ...
0.84737834527845745 ...
0.78347864586745768 ...
0.78347863763547879 ...
...
How the list is sorted does not matter. The only important thing is that it is complete. If we can truly count all of the values, our list must contain every real number between 0 and 1. But Cantor demonstrated that he could construct another number between 0 and 1 that does not appear in the list.
He did it in the following way: the first decimal place of the new number corresponds to the first decimal place of the first number in the list plus one—that is, 4 in the above example. The second decimal place is obtained by calculating the second decimal place of the second number plus one—that is, 5. For the third, you increase the third decimal place of the third number by 1, and so on. (If you hit a 9 you can change the value to a 0.)

To set up Cantor's Diagonal argument, you can begin by creating a list of all rational numbers by following the arrows and ignoring fractions in which the numerator is greater than the denominator. Credit: Buckyball Design; Source Wikipedia
In this way, an irrational number with an infinite number of decimal places will be obtained. It does not appear in the list because it always differs in at least one digit from each number listed. Thus, the list cannot be complete, which contradicts the original assumption. Cantor could therefore conclude that there are “uncountably many” real numbers.
A Provably Unprovable Assumption
Besides the cardinality ℵ0 of the natural numbers, there is at least one more (uncountable) infinity—and it might be a better choice than ℵ0 in the competition to find the largest number.
Just how large is the set of real numbers? Cantor asked himself this same question when he was investigating whether a set of numbers existed that would be larger than the natural numbers but smaller than the real numbers. Finding no such set, the mathematician formulated his famous “continuum hypothesis” in 1878. It states that there is no set whose cardinality lies between that of the natural numbers and that of the real numbers.
But Cantor could not prove his assumption—nor could anyone else. As it turns out, the continuum hypothesis belongs to the statements that elude our basic mathematical framework. They are provably unprovable: you can neither prove nor disprove the conjecture by the usual mathematical means. (Kurt Gödel proved that there is such incompleteness in every meaningful formulation of mathematics in 1931.)
In other words, you could assume the continuum hypothesis to be true and never encounter a contradiction. Conversely, however, you could postulate that there are other infinities between the cardinality of the natural and real numbers—and encounter no problems either. This is not particularly satisfying for mathematicians. After all, we are talking about the size of the real numbers—nobody knows how many of these values exist. In response, some people are trying to extend the basic framework of the subject to derive a tool from this larger theory to prove or disprove the continuum hypothesis.
Experts are by no means united in this effort. The foundation of mathematics, the “Zermelo-Fraenkel set theory,” consists of nine unproved basic statements (so-called axioms) that form a foundation for the entire subject. It took several attempts to find a suitable set of axioms for the task because it must fulfill several requirements. The set should have as few axioms as possible, and they should be intuitively true and not too complicated. Other examples are the axiom of the empty set, which states that a set without elements exists, and the axiom of pairing, according to which two sets with the same elements are equal.
The nine axioms of the Zermelo-Fraenkel set theory (together with the axiom of choice) are sufficient to build up the mathematics that we know. But the continuum hypothesis eludes them. In order to investigate the cardinality of the real numbers in more detail, you must extend the current set theory to include other basic statements. For example, you could append the statement “The continuum hypothesis is true” to the group of axioms. This would not be a good axiom, however. Unlike the other statements, it is not directly obvious why this one should be true.
Therefore, experts are looking for other axioms that are intuitively true and can be used to investigate the continuum hypothesis. There are already a few promising candidates—some would confirm Cantor’s conjecture, while others would refute it. Which extended version of set theory will prevail, if any, remains to be seen. As long as this is the case, the question of how many real numbers exist remains unsolved.
Even if that remains a mystery, it has long been known that there are sets with much larger cardinalities than that of the set of real numbers—even though the real numbers are themselves infinite. And if you want to win the largest number game, you should go chase the largest infinity.
This article originally appeared in Spektrum der Wissenschaft and was reproduced with permission.
