Copper, platinum, aluminum, gold. Metals have been so important in human history that we’ve named historical epochs (the Bronze Age, the Iron Age) for their transformative power. These materials, with their characteristic ability to conduct electricity, are no less vital to our modern era—they are found in essentially every technology that has enabled the information age. Yet despite metals’ utility, scientists are still trying to decipher their inner workings.
After centuries of study, physicists have a good understanding of most metals. Their characteristic glossiness and coldness to the touch are both consequences of the way their electrons move and interact. A metal’s reflective sheen, for instance, comes from its ability to conduct electricity at even the extremely high frequencies of visible light, and it feels cool to the touch as a result of how well it conducts heat compared with an insulating material such as wood or glass.
Recently, though, scientists have discovered that some metals are different. A new class of materials called strange metals shows confusing electronic behavior. In these metals, electrons seem to lose their individual identities, acting more like a soup in which all the particles are connected through quantum entanglement. The physics of these strongly interacting electrons even seems to mirror some of the ways particles act at the event horizons of black holes. By learning about these weird outliers, physicists hope to gain a better understanding of all metals and of the extremes of physics that are possible within solid materials.
On supporting science journalism
If you're enjoying this article, consider supporting our award-winning journalism by subscribing. By purchasing a subscription you are helping to ensure the future of impactful stories about the discoveries and ideas shaping our world today.
To see why strange metals are strange, let’s first consider how regular metals work. Like all physical materials, metals are made of atoms: positively charged nuclei surrounded by negatively charged electrons. Materials are held together by chemical bonds, through which atoms transfer and share electrons. In metals, some of those electrons (“valence” electrons) can move easily across many atoms, flowing freely throughout the material and carrying their negative electric charge with them when propelled by an electric field. This movement is called an electric current.

Credit: Jen Christiansen
Electrons were discovered in 1897, right around the time when physicists were beginning to define the statistical properties of large numbers of particles. Given that a cubic centimeter of a solid contains something like 1022 atoms, any approach to describing real materials needs to be statistical. Early attempts to apply physics to the workings of solid materials assumed electrons in metal behaved like molecules in a gas. By the late 1920s, however, the first quantum revolution had taken place, revealing that electrons, like all quantum particles, have wavelike properties.
By thinking of electrons as waves, we can imagine a quantum toy model of a metal. Each electron wave has a momentum that is inversely proportional to its wavelength and a kinetic energy proportional to the square of its momentum. If we consider just one dimension, we can think of these states as traffic lanes on a highway. The lowest-energy state is the slow lane, and there is an eastbound and a westbound slow lane. A quantum law known as the Pauli exclusion principle, which states that no two electrons can share identical quantum states, implies that each lane can hold two electrons (cars), with one electron taking a value of “spin-up” and the other “spin-down.” (The quantum property of spin represents the electron’s angular momentum but isn’t equivalent to anything actually spinning.)
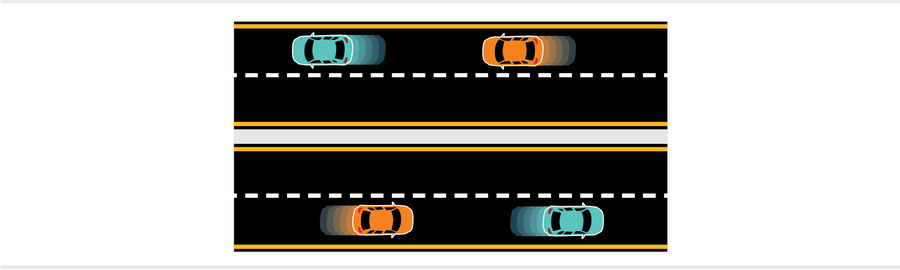
Credit: Jen Christiansen
Once the slow lanes are full, additional electron cars must go into higher-speed lanes. Now imagine many, many electron cars and therefore many, many lanes. The electrons in the slowest-moving lanes are unable to change lanes because the lanes with immediately higher or lower energy are already full (this is called Pauli blocking). Only the highest-speed electrons have access to unoccupied lanes.

Credit: Jen Christiansen
Now hook up a battery to the system to add an electric field pointing west along the highway. This field accelerates the eastbound electron cars so that the electrons with open lanes next to them can shift up to the higher-speed lanes. It also decelerates the westbound cars, causing the electrons with open slots near them to move into lower-speed westbound lanes. The resulting net increase in eastbound traffic (negative charges moving east) corresponds to an electrical current moving west.

Credit: Jen Christiansen
I call this model a toy because it deliberately ignores two important things. First, it describes only the electrons and doesn’t have anything to say about the actual atoms that make up the material. But an amazing thing happens in crystalline solids, in which the atoms line up in regular, repeating lattices. The state of each electron looks like a wave that already takes into account the lattice. Our analogy of car lanes still applies, but now there are bands of lanes (energies) with gaps between them. If the highest-energy occupied lane has empty adjacent lanes, then the system is a metal because lane changes are still possible. In other words, the material can conduct current. If all the lanes in each band are completely full or completely empty, the system is either an insulator or a semiconductor. If it’s an insulator, the energy gap between bands is very large compared with the thermal energy scale (a diamond, for example). If it’s a semiconductor, the energy gap between bands is smaller (such as silicon).
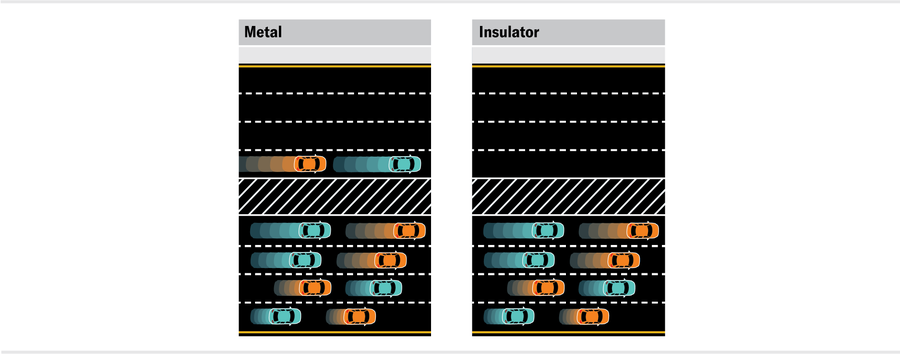
Credit: Jen Christiansen
The second reason this model is a toy is that it ignores interactions between electrons, even though we learn in elementary school that like charges repel each other. The Pauli principle is key to why we can get away with this. In atoms, as this law forces additional electrons to go into higher- and higher-energy states (also called orbitals), the kinetic energy often ends up being more important than the electron-electron repulsion.
Almost 70 years ago Russian physicist Lev Landau and his collaborators introduced an incredibly successful conjecture, now known as Landau Fermi liquid theory, to try to understand electron interactions within metals.
The interacting electrons in a Fermi liquid give rise to what we call quasiparticles. A quasiparticle is an excited state that has all the properties we’d associate with a particle (charge, spin, momentum, energy) except that it exists only when embedded in a larger, many-body system. An analogue of a quasiparticle is the “wave” cheer in a sports stadium. When fans do the wave, an observer can clearly see a pulse of standing people that seems to move around the stadium, at any time having a clear position and speed. The wave is a collective object built out of the coordinated motions of the interacting audience members, and it doesn’t even make sense to talk about the wave when there are only scattered fans—it needs a crowd. Physicists have discovered an entire zoo of quasiparticles in solid materials with names such as phonons, magnons, spinons, holons and plasmons.
Quasiparticles are the result of electron-electron interactions. An analogy would be a person trying to make their way through a crowded room—their speed, for example, is not what it would be in an empty room (the noninteracting case), because their motion is affected by the other people, who have to rearrange themselves for the person walking to get through.
By thinking of the collective actions of electrons as quasiparticles, physicists have made testable predictions that have been verified time and again in experiments on metals such as gold, silver, copper and aluminum. For example, the electrical resistivity—how much a material resists the flow of a current through it—of a Fermi liquid at low temperatures is predicted to vary in proportion to the square of the temperature, and experiments show that it does.

Credit: Jen Christiansen
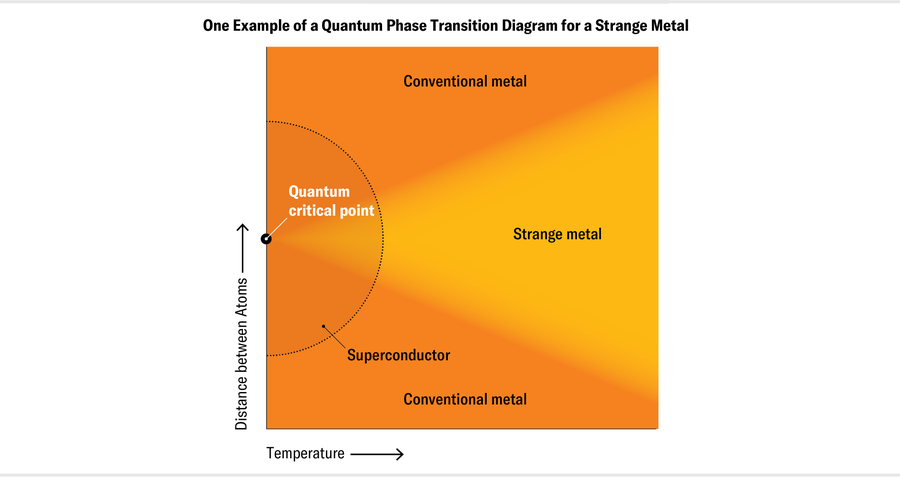
In recent years physicists have found a dozen or more materials that are clearly metals, in the sense that their electrical resistivity decreases with decreasing temperature, but that are not Fermi liquids. These “strange metals” have resistivity at low temperatures that is linearly proportional to temperature—that is, their resistivity varies with the actual temperature rather than with the square of the temperature. Examples of these materials include some copper oxide superconductors, some iron-based superconductors, some “heavy fermion” materials, and twisted bilayer graphene under certain conditions.
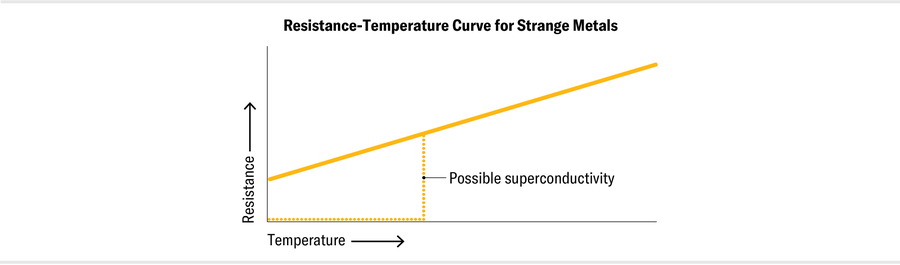
Credit: Jen Christiansen
Most of these arose from laboratory experiments looking at materials known to be near a transition between phases (such as between a superconductor and a metal or between different magnetic states). At first glance, these strange metals don’t appear very remarkable. Although they tend to be hard and brittle rather than soft and ductile, their “strangeness” is not apparent at room temperature, because thermal energy swamps the quantum effects when these materials are warm. Many materials seem to be perfectly ordinary metals until they are near a particular quantum critical point, in terms of temperature, pressure, and other parameters. At this critical point, if the materials are near absolute zero (in their ground state), they switch between two different phases—for example, two ordinary metals with different magnetic properties. At higher temperatures and energies, they turn into strange metals.
Credit: Jen Christiansen
Whether the resistivity depends on T or T2 at low temperatures may sound like an innocuous difference, but it’s not. This change implies the failure of Fermi liquid theory and, some scientists think, the breakdown of the quasiparticle picture of excited electrons. In the absence of quasiparticles, it’s as if the electrons lose their individuality and act collectively as a strongly interacting soup in which all the particles are highly entangled. Entanglement is a kind of quantum connection that makes particles’ fates intertwined. And when the electrons within a metal become strongly entangled, their collective behavior changes.
One fascinating outcome is that the timescale on which the electrons distribute momentum among themselves in many (but not all) strange metals is “Planckian,” meaning it is essentially governed only by quantum mechanics (through what’s called Planck’s constant) and temperature, independently of any details of the materials. This kind of universality among all strange metals, and the fact that strange metallicity appears in many different materials, suggests there is some deeper organizing principle at work. Theoretical models describing strange metal phenomena can tend toward the exotic. Some models even map the electron behavior to the physics of event horizons around black holes.
This is the kind of fun challenge that inspires experimentalists. What measurements can we do, beyond resistance versus temperature, to see what’s going on microscopically in strange metals? How can we tell whether the electrons are acting cooperatively in some weird quantum soup or as nearly independent electronlike quasiparticles? There is a puzzle-solving aspect of experimental physics: How can we use a limited set of tools to distinguish experimentally between different models of what might be going on, given that we can’t watch every electron?
In my view, there are four particularly promising experimental avenues for probing strange metals. One approach involves using a beam of electrons to deliver precise amounts of energy and momentum to the electrons within the metal, a process called momentum-resolved electron energy-loss spectroscopy. By mapping out how the entire electronic system can absorb energy and momentum, physicists can distinguish between contributions from conventional quasiparticles and those from a collective quantum response.
A second technique relies on recently developed, very precise methods for studying how a current flows within a material. In this approach, physicists employ incredibly sensitive magnetic field sensors. One sensor is a superconducting ring (a superconducting quantum interference device, best known as SQUID) that generates a voltage precisely related to the amount of magnetic field that flows through the ring. Another sensor is made of a diamond crystal with a particular defect—a nitrogen atom next to a spot where there should be a carbon atom but isn’t (a “nitro-gen vacancy center”). Its optical properties are quite closely linked to the local magnetic field, making it a good field sensor. By mapping out the magnetic fields near a strange metal, researchers can infer the detailed local flow of current, including signs of electrons cooperating, with the sea of electrons acting like a fluid with very low viscosity.
A third method uses optics techniques to investigate the transmission and reflection of light with wavelengths between about 100 nanometers and one millimeter. Researchers use this approach to infer how the electrical conduction process, including scattering, depends on the frequency of the electric field. The relations among conductivity, frequency and temperature can give greater insights into whether the material is Planckian.
The fourth approach, which we use in my lab, is to measure “shot noise” in the current flowing through a strange metal. Shot noise is related to the granularity of electronic charge. If a current is carried by discrete charge carriers (such as individual electrons or quasiparticles), as opposed to a continuous soup, statistical variation in their arrival times leads to fluctuations in the current. Consider raindrops falling on your roof. The same amount of water per unit time can arrive as a smooth, steady rain of many small drops or as a strongly fluctuating shower with fewer but much larger drops. Measuring the current fluctuations gives us information about how much charge is transported at a time.
Our measurements are possible only because we use high-quality films of one strange metal, a compound with the chemical formula YbRh2Si2, developed by our collaborators at the Technical University of Vienna in Austria. This material has a quantum critical point between two distinct Fermi liquid states that becomes clear and sharp at temperatures less than 50 thousandths of a degree above absolute zero, depending on the magnetic field. In my lab, we created nanoscale wires from these films and watched the shot noise in the current. At the temperatures of our experiments, three kelvins up to 10 kelvins, this material is very much in the strange metal regime. If the electrons in this wire flowed more like a continuous fluid rather than a stream of discrete quasiparticles, the shot noise would be lower than that of a Fermi liquid. We did in fact measure a suppressed shot noise in our strange metal, whereas control measurements on gold wires showed the expected Fermi liquid results. Our measurements don’t yet line up with a definitive theoretical model for what’s going on in strange metals, but they help to point us in the right direction for one.
Strange metals present an apparent failure of one of the most successful physics models of solids. For physicists, this failure is a great temptation for exploration. Yet these metals are not just a topic of purely curiosity-driven basic research. They could potentially be important for urgently needed technologies. Scientists have observed superconductivity emerging in multiple families of strange metals at relatively high temperatures. Understanding these metals may help us develop superconductors that might operate at or close to room temperature, potentially transforming power grids, quantum computing and medical devices. If our explorations of the strange frontiers of solid-state physics are successful, there is always a chance that the next epoch of technology will come to be known as the Strange Metal Age.